K.M. Borkowski

Toruń Radio Astronomy Observatory, Nicolaus Copernicus University,
ul. Chopina 12/18, PL-87-100 Toruń, Poland

Received July 4, 1983; accepted August 9, 1985

Summary. The analysis presented in this paper shows that the usual small signal approximation in VLBI is acceptable in the majority of applications. However, it leads to nonlinear systematic overestimation of the correlation coefficient, which in some cases cannot be considered negligible. For example, the correlation of about 0.26 estimated from the fringe amplitude of 0.1, as measured with the Mark II system, is 3.4% too high. Formulae suitable for accurate conversion of measured amplitudes into the correlation coefficient for a few useful fringe rotation schemes are given. The analysis also brings evidence for a small bias in the phase measurements made with present day VLBI correlators, which is entirely due to imperfect fringe rotation. Arguments are presented in favour of five-level rotators. Key words: VLBI observables – data analysis – instruments 1. Introduction Very long baseline interferometry (VLBI) is a relatively new powerful technique for studying radio source structures, their positions and baseline orientations with angular accuracies as high as a fraction of milliarcsecond and in special cases approaching the microarcsecond level. The high resolution and sensitivity of this technique may be easily degraded by imperfect signal processing. By comparison with results obtained with the MERLIN, Wilkinson (1983) has demonstrated that the dynamic range of VLBI maps is considerably less than might be expected. In fact it is by no means a rare event that VLBI users report the presence of unknown sources of errors in their data. Factors that place limits on accuracy of VLBI measurements are numerous and generally well documented in literature. Here we shall concentrate on two which received little, if any, attention and which result in relatively small but systematic baseline related errors. One of them arises with the use of a small signal approximation to the Van Vleck relation when determining the correlation coefficient from the fringe amplitude measurements made with digital VLBI systems. In effect the usual signal processing leads to somewhat biased estimates of the correlation. The other seemingly overlooked source of biases, this time affecting also the measured phase of fringes, results from the use of an imperfect fringe rotation in VLBI correlators. This problem is less fundamental and can be dealt with by improving the correlation process. A correlator with a five-level fringe rotator would perform much better in this respect, offering in addition considerably smaller losses in the signal to noise ratio (hereafter SNR).

2. Expected fringes The process of correlation of VLBI data involves a fringe rotation, or fringe stopping, that is the multiplication of one of the two data streams by an approximation to a sinusoid whose frequency is set at a predicted rate of the fringes. The fringe rotation and correlation of two binary data streams, xi and yi, yield the stream of xiyi FRF(φi), (1) where FRF stands for the fringe rotation function and φi is the predicted phase of the fringes for i-th data pair. This stream is averaged to improve the initially poor SNR. The expected value of the averaged correlator output at its cosine channel is thus: rc = ΣiρiFRF(φ i) / Σi|FRF(φi)|, (2) where ρi is the statistical expectancy of the product xiyi. A similar expression may be written for the sine channel to give rs equal to the right side of (2) with the φi replaced by a φi – π/2. The Van Vleck relation (Van Vleck, Middleton 1966) is ρ(φ) = 2 π arcsin[r(φ)], (3) where r is the correlation coefficient of the original (unclipped) signals with its true phase φ. Now it is important to observe that the r is not a sinusoid, and that the expected SNR after correlation of the original signals may be expressed by μ = μo|cos(φ)|, (4) where μo = ro/(1 – ro), and ro is the amplitude of the r. Taking the sign of the fringes into account allows one to reverse (4) and finally write r(φ) = ro cos(φ) 1 – ro[1 –|cos(φ)|] , (5) which, when introduced to (3), gives a model for the expected infinitely clipped fringes. The output of the digital VLBI correlator is thus expected to have the form of (2) with ρi being the samples of such fringes.

r(φ) = ro cos(φ),

(6)

which is followed by another approximation,

ρ(φ) = 2 π rocos(φ),

both of which are obviously good for very small r_o. Note, however, once again that variations in signal transform nonlinearly into variations of the correlation coefficient.

Neglecting the difference between (5) and (6) already gives rise to an error. The infinite clipping leads to another nonlinear transformation — that expressed by Eq. (3). These, already serious, problems with analytical treatment of the expected correlator output are still further complicated by the approximation of the FRF. Nevertheless, the output can easily be predicted numerically using Eqs. (2), (3) and (5). The results of such an approach are presented in Sect. 4.

3. Approximated outputs

In order to check the validity of the results to be presented in the next section and to introduce our notation here we first recall the commonly used small signal approximation and then derive a large signal approximation.

With small signals, r_o ≈ 0, the sampled form of Eq. (3) may be safely approximated by

ρi = 2 π rocos(φi – φo).

(7)

For a large number of averaged samples, which is almost always the case in VLBI practice, the sums in (2) may be approximated by the integrals. Taking now for the FRF a pure sinewave one obtains

rc = ro 2 cos(φo).

(8)

In case of the three-level FRF, which is a squarewave zeroed over intervals of length π – 2α centred at each of its zero-crossings, the result of integration is

rc =  2 π ro  sinα α cosφo.

(9)

In both these cases the sine channel output gives r_s(φ_o) = r_c(π/2 – φ_o).

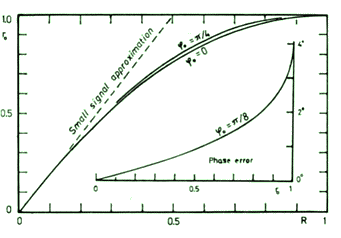
It is clear from the above that to calculate the correlation coefficient, r_o, from the fringe amplitude measurements, R = (r_s² + r_c²)^1/2, a proportionality factor of r_o/R is needed. It equals 2, or (π/2)α/sinα when the unquantized sinewave, or the three-level FRF, respectively, are used. The factor α/sinα appears in literature under the numerical value of 1.28 corresponding to α = 3π/8 (e.g. Reid et al., 1980). The fringe phase, determined as Φ = atg(r_s/r_c), appears here as the unbiased estimate of the true phase φ_o.

When a signal is very large, i.e. the correlation coefficient is very close to 1, the Van Vleck relation reduces to

ρi = ro sgn[cos(φi – φo)], ro ≈ 1,

(10)

and the expected correlator output at the cosine channel becomes

rc = rocosφo

(11)

for the sinewave FRF, and

rc = ro, |φo| ≤  π 2 – α ro α ( π 2  – |φo| ) ,    π 2 – α ≤ |φo| ≤ π 2

(12)

for the three-level FRF. Outside the ±π/2 range of phase these outputs possess the cosine function periodicity with the symmetry r_c(φ_o) = –r_c(π – |φ_o|). The relation r_s(φ_o) = r_c(π/2 – φ_o) now holds also true.

The amplitude of the fringes is here twice that of the small signal approximation in the sinewave FRF case: R = r_o. For the three-level FRF it is now also phase dependent and has the magnitude

R = ro α √   φo2 + α2   , |φo| ≤ π 2 – α ro α √ (  π 2 – |φo| ) 2   + φo2   ,     π 2 – α ≤ |φo| ≤ π 4

(13)

and the period of π/2. The fringe phase estimate is no longer equal to the true phase of the fringes and the difference is

Φ – φo = atg(φo/2) – φo, |φo| ≤ π 2 – α atg   φo π 2 – |φo| – φo,      π 2   – α ≤ |φo| ≤   π 4   .

(14)

This difference, as a function of φ_o, also has the period of π/2. It should be noted that with α = 3π/8 the extreme deviation in the expected phase observable (14) is reached at points where φ_o is an odd multiplicity of π/8, and only slightly exceeds 4° in the absolute value. Further analysis shows that this is also the maximum deviation over the entire range of the r_o.

The formulae presented in this section for the three-level FRF apply also to the two-level FRF, for which α = π/2. Similar analyses are easily carried out for higher-level quantized FRFs.

4. Accurate outputs

In general, the VLBI signals to be correlated are neither small nor large enough for the considered approximations to be used for calculation of the true observables without introducing biases. We have computed the expected outputs, for a number of r_o values, using the complete model for fringes (see Sect. 2):

rc= 2 π Σi arcsin  rocos(φi – φo) 1 – ro[1 – |cos(φi – φo)|] FRF(φi) Σi|FRF(φi)| .

(15)

About 2000 equidistant samples taken over one cycle of the model fringes were thus averaged with the help of the R-32 computer (IBM 360 near equivalent), to yield the correlator responses for each set of the initial fringe parameters (i.e. r_o and φ_o) and for a few different FRFs. Some of the numerical results are presented in Table 1. The results, whenever appropriate, were found to agree within five significant digits with the analytical results obtained for the small and large signal approximations as derived in the previous section. As could be expected, the output amplitude and phase biases, if they exist, display the π/2 periodicity with respect to, and are even functions of, the true phase.

Table 1. Expected correlator output amplitudes (R) for two fringe rotation functions (FRF) and some fringe phases, φo, as a function of the correlation coefficient, ro

Sine Three-level FRF ro FRF ----------------------------------- μo φo = 0 φo = π/16 φo = π/8 φo = π/4 0.001 5.002E-4 4.993E-4 4.993E-4 4.993E-4 4.993E-4 0.001 0.01 5.008E-3 5.001E-3 5.001E-3 5.000E-3 4.999E-3 0.010 0.1 0.05086 0.05087 0.05085 0.05078 0.05065 0.111 0.15 0.07703 0.07713 0.07708 0.07699 0.07663 0.176 0.25 0.1313 0.1317 0.1316 0.1312 0.1303 0.333 0.35 0.1888 0.1897 0.1895 0.1887 0.1869 0.538 0.55 0.3184 0.3209 0.3206 0.3188 0.3132 1.222 0.75 0.4835 0.4884 0.4886 0.4863 0.4715 3.000 0.85 0.5950 0.6009 0.6035 0.6012 0.5764 5.667 0.90 0.6668 0.6729 0.6758 0.6765 0.6431 9.000 0.95 0.7622 0.7677 0.7731 0.7787 0.7303 19.000 0.99 0.8926 0.8957 0.9055 0.9249 0.8476 99.000 0.999 0.9661 0.9670 0.9796 1.0120 0.9127 999.000 1.000 1.0000 1.0000 1.0139 1.0540 0.9428 ∞

When the unquantized sinewave is used as the FRF, the correlator output amplitude R depends only on the input correlation coefficient r_o, and not on the phase φ_o. Using the least squares fit to some data points it was found that the correlation coefficient can be determined from measurements of the amplitude through the following approximation

ro = sin[2R(1 – 0.294 R + 0.08 R2)],     0 ≤ R ≤ 1

(16)

with an accuracy of 0.05%. The expected fringe phase Φ for such a correlator is error free, i.e. it is equal to the true phase φ_o.

Correlators with other FRFs produce outputs that depend on both, the correlation coefficient and the fringe phase. In case of optimally designed three- and higher-level correlators these outputs are very close to the output of the sinewave FRF correlator. The closeness is such that the formula (16) may be used always, whenever errors not exceeding 2% of r_o are acceptable, regardless of actual phase. The following formula represents somewhat better fit for the common three-level correlator:

ro = sin[2.003R(1 – 0.296 R + 0.1 R2)],

(17)

which departures from the true values less than 1% below r_o = 0.5, and less than 1.5% elsewhere, for all phases. This fit cannot be made much better without accounting for the phase dependence of the real output. The phase measurements for this correlator are in systematic error. The error (see insert in Fig. 1.) falls monotonically from about 4° at r_o = 1, to 1° at r_o = 0.5, to vanish at r_o = 0 (these are the maximum values as the φ_o is varied).

A near optimal correlator with a five-level FRF (α = 13π/30 plus doubling the x_iy_i product over the interval of 7π/15 placed at the center of each nonzero interval of the three-level FRF) would have still closer response to that with the ideal FRF. The measured phases would differ from the true phases by no more than 0.1° for r_o < 0.65, and by less than 0.5° for r_o < 0.99. The following approximation

ro = sin[2R – (3 – R)R2/5]

(18)

Fig. 1. Relationship between the expected fringe amplitude, R, and the correlation coefficient, ro, for a correlator with the three-level fringe rotation function, and for the phases φo = kπ/2 (lower curve) and φo = (2k + 1)π/4, where k is any integer. Inserted is the plot of the maximum expected absolute error in the phase determination (in degrees) for the same correlator and the phase φo = (2k + 1)π/8

would be applicable for such a correlator within the tolerance of deviation of 0.3% of r_o for r_o < 0.6, and for all phases.

5. Discussion

VLBI signal processing normally introduces various corrections to the measured observables. The nonlinearity of the correlator response analysed in this paper rises practical question: to which quantity a particular correction should be applied? Besides the Van Vleck correction and the one due to the shape of an FRF, which are explicitly included in Eqs. (16) through (18), there are three other categories of corrections: those that apply to (1) the measured amplitude — R; (2) the correlation coefficient — r_o; and (3) the signal to noise ratio — μ_o = r_o/(1 – r_o). In our opinion the first category should include factors due to the following effects: smoothing caused by averaging of not exactly stopped fringes, loss of quadrature between channels, and bias (nonzero mean) of the clipper-sampler (this loss is proportional to the sum of the absolute values of the biases in the two data streams, assuming the biases are small). Other effects, such as those due to the finite coherence time of interferometer, the delay tracking by integral bit shifts, or the aliased noise from outside the standard frequency band, belong either to the second or to the third category depending on the way the numerical factors were derived.

With the Mark II system a composite correction factor of about 2.6 is used to convert the measured amplitudes into the correlation coefficient. From this value, according to our distinction, only a factor k = 1.3 remains to be applied to (17) to make the formula practically applicable:

r^o ≈ 1.3 sin [2.003R(1 – 0.296R + 0.1R2)].

(19)

Though in the above it was assumed that the k factor applies fully to the correlation coefficient, the multiplicatory coefficient related to the signal to noise ratio (μ_o) can, if required, be portioned out of it and applied separately to r^{^}_o/(1 – r^{^}_o) to yield the final μ^{^}_o.

With assumption of small signals the Eq. (19) is reduced back to the commonly used approximation

r^o ≈ 2.6R.

(20)

Fig. 2. Expected overestimation in percent of the source flux density, or input SNR, (as determined from fringe amplitudes obtained with the three-level fringe rotation function), plotted against the correlation coefficient. The lower curve corresponds to the fringe phase φo = (2k + 1) π/4, where k is any integer

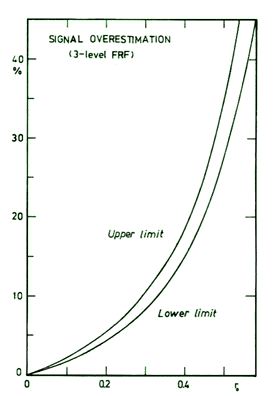
The quality of this approximation to the correlation coefficient can now be easily judged by comparison of the last two equations. While for very small amplitudes the difference is evidently negligible, it becomes more and more significant as the signal grows. More convincing insight is given by Fig. 2., which illustrates the effect of neglecting the nonlinearity on the estimates of the SNR, μ_o (or corresponding ratio of antenna temperature to system temperature), which is essential for the flux density scale calibration. The plotted error, the overestimation, has been calculated as the difference between the small signal approximated μ_o and the true μ_o, in percent of the latter. Note that an error in μ_o is always greater than the corresponding error in r_o, although for small signals the difference is again negligible.

In practice, with a pair of large telescopes and reasonably good system temperatures, the correlation coefficient, ro as in (17), of 0.1 may be exceeded for sources of the flux density of only a few Janskys. At this level of correlation the discussed overestimation of flux density is already about 2%. Consequently, if such a source is taken for the calibrator, then all fluxes ofweaker sources are underestimated by up to 2%. The dependence of this error on actual flux will cause distortions in maps of strong sources because different baselines produce different correlations being a function of the source visibility and the telescope sensitivities.

As a result of imperfect fringe rotation the phase of the measured correlation coefficient is also slightly biased. This bias is, however, of negligibly small magnitude for practically all astrophysical applications of VLBI (with the three-level FRF it is smaller than 1° for r_o < 0.5). Anyway, it is worth remembering that the resulting errors do not close in the closure phase observable. In applications which require very precise phase measurements, such as in astrometry, geodesy and navigation, this phase bias may be a source of appreciable errors, especially when strong artificial sources are used. Such errors again do not cancel in differential observables, because they depend upon the actual fringe phase on a given baseline. Note, for instance, that the phase error as small as 0.1° on a 10⁶ λ baseline is equivalent to the apparent source position offset of about 60 microarcseconds.

Having in mind the inconveniences caused by the imperfect three-level fringe rotation we have analysed also the properties of a five-level correlator, which differs from the former only in that it requires the doubling of the correlation product around the extrema ofthe corresponding sine rotation function. The analysis showed that the phase bias here is reduced about 10 times, as compared to the three-level FRF case. Additional study, not presented in this paper, proves also that the five-level correlator would introduce 3 times smaller losses in SNR than does the three-level correlator. To emphasize this point, note that the 4% loss in SNR of the presently used correlators is equivalent to the dropout of about 8% of recorded data, i.e. roughly one hour in every 12 hours of observations. With the five-level correlator this loss reduces to about 20 minutes only.

6. Conclusions

Presented analysis of the digital radio interferometer response shows that the output amplitude of fringes is in general a highly nonlinear function of the strength of an input signal as expressed by the correlation coefficient, or the signal to noise ratio. The cause of this nonlinearity lies in the behavior of the clipper-sampler embodied in the Van Vleck relation. Further complication is due to the quantization of the lobe rotation function which introduces a phase dependence to the correlator output.

In case of the three-Ievel fringe rotator, which presently is almost exclusively used in digital VLBI correlators, the nonlinearity, if neglected, produces a bias (overestimation) in the signal power determination. The bias is smaller than 2% if the correlation coefficient, r_o, remains below about 0.1 or, equivalently, the measured output amplitude of the fringes is less than 0.055 for the Mark II and Mark III system. This error grows quickly for greater amplitudes (see Fig. 2.). The normal signal processing fails to account for this nonlinearity. In fact, the usual small signal approximation breaks down completely near the center of the output amplitude range. The analysis revealed also a small bias in the phase of the fringes as measured with the use of the three-level fringe rotation function. Its magnitude is smaller than 1° for r_o < 0.5 (see insert in Fig. 1).

For three fringe rotation schemes of practical interest approximate, relatively simple formulae are presented [Eqs. (16) through (18)] that allow one to calculate the correlation coefficient with biases reduced to less than 1.5% for all measured amplitudes and phases.

Considering the drawbacks of the three-level correlator, arguments were put forward in favour of a five-level correlator. Such a correlator has the potential of not only almost eliminating the fringe phase influence on observables, but also of reducing the signal to noise ratio losses by a factor of 3, i.e. from 4% to about 1.3%.

Finally, the placement of various correction factors in signal processing was discussed. Distinction was made between effects that cause losses in the fringe amplitude as measured by a VLBI correlator, in the correlation coefficient, and in the signal to noise ratio. This was necessary because, in view of the analysed nonlinearity, a correction of each of these quantities has in general a different effect on the final result.

Acknowledgements. The author thanks Dr. A. Kus and Prof. S. Gorgolewski for numerous discussions and help in preparation of this paper. Thanks are also due to Dr. D. Graham who as the reviewer suggested to combine two earlier papers originally submitted to this Journal in July 1983 and April 1984 which, along with other criticisms, resulted in this improved version.

References

Reid, M.J., Haschick, A.D., Burke, B.F., Moran, J.M., Johnston, K.J., Swenson, G.W.: 1980, Astrophys. J. 239, 89

Wilkinson, P.N.: 1983, in Very Long Baseline Interferometry Techniques, CNES, Cepadues Editions, Toulouse, p. 375

Van Vleck, J.H., Middleton, D.: 1966, Proc. IEEE 54, 2