1 Introduction
Among those who studied calendar structures the opinion seems to prevail that our Gregorian calendar, introduced in 1582, although not perfect, does not require any revision as to its year length or rules of intercalation of leap days, still for thousands of years to come. Quite a usual approach to reach such a conclusion is a comparison of the mean length of the tropical year (taken either as a constant unit or one linearly diminishing) with the mean length of the calendar year, combined with certain summation of both. In the present study I shall demonstrate that the approach is methodologically incorrect, in spite of about correct results it normally yields.In his recent article Peck (1990) has detaily analysed a possible reform of solar calendar in view of the changing length of the tropical year. Unfortunately, his ideas are based on wrong grounds and not only because of the mentioned incorrect approach. This author has also overlooked the limited time span the Newcomb formula is valid over and, equally importantly, has ignored the variable Earth rotation. My analysis shows that our present knowledge allows to plan solar calendars roughly for 2-3 thousands of years into the future, and going considerably beyond this range remains a pure speculation carrying little, if any, practical significance. Specifically, the Gregorian calendar rules, used now for over 400 years, serve their purpose very satisfactorily and will do so still for at least a thousand years or so.
2 Longitude of the Sun versus solar calendar
The natural basis for computing passing tropical years is the mean longitude of the Sun reckoned from the precessionally moving equinox (the dynamical equinox or equinox of date). Whenever the longitude reaches a multiple of 360° the mean Sun crosses the vernal equinox and a new tropical year begins. In a modern theory of the motion of Earth around the Sun, VSOP82* (Bretagnon 1982), the mean longitude of the Sun referred to the dynamical equinox is given by (see also e.g. Connaissance des Temps 1990)
| (1) |
| (2) |
As we shall soon see, the equation (1) is entirely sufficient to meet the demends of calendrical calculus. To assess its accuracy and range of validity one may refer to the Laskar (1986) paper where coefficients of polynomials of degree 10 in T are given for the longitude of the Sun referred to a fixed equinox, L′, and for the precession in longitude, pA. The longitude referred to the equinox of date, in which we are now interested, is obtained as L = L′ + pA. In the same paper we read that this more accurate expression is valid (within a few arcseconds) over 10 000 years. In Fig. 1 we have plotted the difference between the mean longitudes of the Sun calculated from the equation (1) and from the Laskar formulation. The reader may now discover how little the VSOP82 expression errs for remote epochs. While in the year 6000 (T = 40) equation (1) yields longitudes too small by only about 1′ and in the year 12 000 (T = 100) the error is still on the order of 0.6°. However, further millenia bring about a rapid change. Forget not that so accumulated error of 1° corresponds to nearly 1 extra day. Since the time-honored formula of Newcomb differs but little from the modern equation (1), we clearly see how dangerous it is to extend its use beyond the range of 10 000 years. In fact, it is also risky to use Laskar's formulae over considerably more than 10 000 years, the range they were designed for. The following discussions will be limited to a few thousands of years in which equation (1) does not introduce any significant errors.
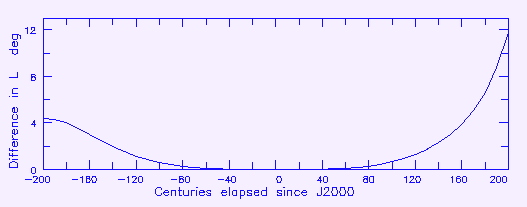 |
Fig. 1. The difference between the mean longitude of the Sun defined in the French ephemeris (equation (1)) and in Laskar (1986). It shows that our third degree polynomial approximation adopted from the VSOP82 theory is very acceptable over a period of about 4000 years from the present. The longitude taken from Newcomb's theory behaves similarly to the one from the VSOP82, so much that at the scale of this figure they would not be distinguishable. |
Through skipping of the constant term in equation (1) and dividing the
numerical coefficients by 1 296 000′′,
the number of seconds of arc in the complete revolution through 360°,
we obtain a convenient expression for
finding the number of rotations of the Sun around the ecliptic, i.e. the number
of tropical years elapsed between J2000 and a given epoch (T):
| (3) |
| (4) |
Now, the difference between counts of tropical (equation (3)) and calendar
years (equation (4)) can be expressed in days by multiplying Lτ – LC by 365.2425, the number of days in the calendar year,
| (5) |
The above considerations assumed a constant duration of a calendar day,
equal to the ephemeris day of 86 400 atomic seconds. We know, however, that
the Earth rotates nonuniformly on its axis and the duration of the calendar or
cilvil day slowly but systematically lengthens. The true number of civil days
elapsed between J2000 and an epoch given by T is the count of rotations of
the Earth:
|
| (6) |
The real problem is that presently we are unable to accurately predict the
duration of the day even for a moderate future, not speaking about thousands
or millions of years. Besides many regular components, the rotation of our
planet exhibits irregular variations on different time scales.
Many researchers attempted to fit a parabola to the measured ΔT
values in order to determine the magnitude of deceleration of the Earth
rotation. The results, when taken together, are rather discouraging.
It is not unusual that formal errors of idividual determinations are on the
order of 1 s/cy2, in contrast to much greater differences between obtained
decelerations themselves.
One of the most recent compilations of determinations of
ΔT based on telescopic observations (McCarthy, Babcock 1986)
leads to the following formula (in seconds of time)
| (7) |
| (8) |
Numerous other results generally fall between these two. Not knowing the true value of the deceleration, to proceed further in our analyses of the future calendars we may temporarily assume the Earth will rotate so that ΔT will lie generally between ΔTMB and ΔTSM. In that case in the year 4000 (T = 20, ΔT between about 6000 and 28 000 seconds) we would have 0.8d < N < 1.1d instead of the earlier calculated N′ = 0.74d. This result alone entitle us to state with confidence that our Gregorian calendar will not deviate from the exact solar calendar by significantly more than 1 day yet for some 2000 years to come.
An estimate made in a similar manner for 10 000 years from the present (T = 100; see Fig. 2) is much more disappointing: 8d < N < 12d. This uncertainty of 4 days, together with quite satisfactory behavior of the Gregorian calendar during one or two nearest millenia, renders approaches to a calendar reform both premature and unnecessary. Should our civilization survive probable social, scientific and technical revolutions of that far future it might for long had forgot about the desire to exactly synchronize its calendar with the Christian Church festivals (recall that the Gregorian reform aimed to fix the date of Easter in accord with the religious tradition). Predicting calendar rules further than several thousand years into the future is a task worth contrary speculation suggesting that future generations will control the Earth rotation to preserve proper agreement of a current civil calendar with astronomical phenomena.
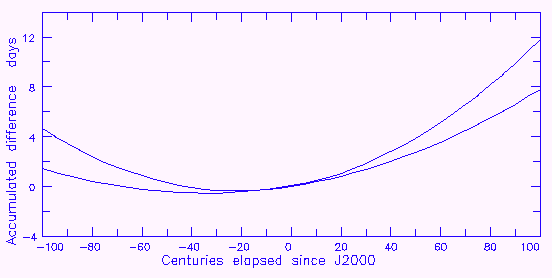 |
Fig. 2. The difference between counts of tropical years and Gregorian years calculated according to equation (6). The lower and upper curve corresponds to supposedly extreme scenarios of Earth's rotation expressed by equation (7) and (8), respectively. |
3 The length of tropical year
Peck (1990) observes a somewhat curious lack of an explicit formula for the length of tropical year in the literature since the introduction of the new system of astronomical reference frames and constants. Using available astronomical literature I myself tried to locate an explanation on how to derive such an expression. Since none has been found in the following we shall derive one.
The tropical year is an orbital period like any other of the many periods met
in astronomy and associated with orbiting celestial bodies. It is
essentially the reciprocal of the mean motion of the Sun.
Thus, in general, if the mean longitude of the Sun can be expressed as
| (9) |
| (10) |
| (11) |
Now, if we take the longitude of the Sun from the Newcomb theory
(a = 129602768.13′′,
b = 1.089′′, c
= 0) we easily obtain his widely known formula (that formed the basis of Peck's paper)
|
Using coefficients of equation (1) in equation (11) we arrive at
a more accurate and up-to-date expression for the length of the tropical year:
| (12) |
Also, it is apparent now that the meaning of equation (12) is that the absolute length of the year is changing. The change is certainly not due to a frictional retardation of the Earth axial rotation, as Hope (1964) has convincingly suggested.
4 The summation of tropical year length is incorrect
In some calendrical discussions, to find the number of tropical years in a given period the summation of the lengths of tropical years is employed. Though the numerical results will be usually acceptable this is principally incorrect method and works only because the length changes very slowly indeed. To substantiate this statement suppose we rewrite our formula for longitude as L = t + ε(t), where t is the time expressed in years of constant duration (e.g. 365.25 ephemeris days). Then the difference of time measured in tropical and constant years is
| (13) |
| (14) |
| (15) |
It should be born in mind, however, that the exactness of |N′′| depends on smallness of the time derivative of ε(t), so that in general it is to be preferable to use directly the mean longitude of the Sun instead of the tropical year length.
5 Conclusion
The general formulae that Peck (1990) has derived and that he has found useful in practice, may be further generalized to conform to the methodologically correct approach and to the more accurate expression for the motion of the Sun. Clearly, the quantity n(T) – 365·Lτ(T), ie. the difference between the total number of days and the count of days contained in Lτ 365-day years, represents the desired number of leap years in a solar calendar between J2000 and the epoch T. Thus, using Peck's Year Zero, AD 0 (JD = 1721058, To = –730487/36525), as the origin for counting years we easily obtain a formula that allows to find the number of leap years until epoch T, necessary in order that given calendar closely agrees with the Sun's motion:
|
| (17) |
The equation (17) can be directly compared with
the equation (5) in Peck (1990):
| (18) |
To sum up, in this paper we have shown or observed that:
K.M. Borkowski
Toruñ Radio Astronomy Observatory,
Nicolaus Copernicus University,
ul. Chopina 12/18,
PL-87-100 Toruñ,
Poland
| REFERENCES |
Connaissance des Temps, 1990, Éphémérides astronomiques 1991, Bureau des Longitudes, Paris.
Hope, E.R., 1964, J. Roy. Astron. Soc. Can., 58, 3.
Laskar, J., 1986, Astron. Astrophys., 157, 59.
McCarthy, D.D., Babcock, A.K., 1986, Phys. Earth Planet. Interiors, 44, 281.
Peck, P.A., 1990, J. Roy. Astron. Soc. Can., 84, 14.
Sôma, M., Aoki, S., 1990, Astron. Astrophys., 240, 150.
Stephenson, F.R., Morrison, L.V., 1984, Phil. Trans. Roy. Soc. London, A313, 47.
Footnote:
*I have often seen the letters "VSOP" on the side of a bottle of wine and I have wondered what the letters stand for. I understand that, in the context of this paper, VSOP82 refers to the 1982 version of Bretagnon's "Variations Séculaires des Orbites Planétaires". Whether this is what is meant on wine bottles remains a mystery to me. — Editor
 |
File translated from TEX by TTH, version 3.12 on 24 Aug 2002.