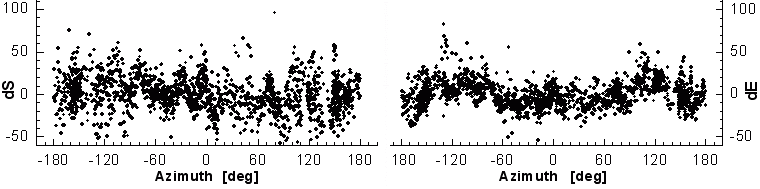TPOINT versus Torun pointing Model4c
| This page documents some analyses
done by Patrick Wallace and me (Kaz Borkowski) in February 2010 with regard
to Torun RT32 pointing modeling in preparation for possible use of the
TPOINT
application for precise Torun telescope pointing as required by the
OCRA program.
It consists mainly of somewhat edited e-mails we have exchanged that time. The first
mail (right below this intro) presents the original data used in our analyses, then there are excerpts of mails containing some of Patrick's results followed by more details
about my analyses of residuals from fitting of the
Model4c,
and the last mail is a summary presented to the OCRA team.
With the proviso that the available observations contain
unresolved anomalies and may themselves be limiting the
model fitting, our analyses of the residuals show comparable
results using either my program or TPOINT, both based on analytical
models. TPOINT provides a more versatile modelling environment,
with a repertoire of built-in pointing terms and a range of
graphical presentations, and its models can readily be
integrated with a telescope control system; it is also widely
used, whereas my own program is a purely in-house facility.
The latter property does, however, have some advantages, in
particular that the code can be extended (for example to add
special-to-Torun pointing terms) and that there is provision for
weighted fits.
— Kaz
Torun, May 11, 2010 |
|
Subject: Re: 32m pointing
From: "Kazimierz Borkowski"
Date: We, February 10, 2010 14:47
Dear Patrick,
(...)
In my work with these data I never used meteo data, but they are
there almost ready to use. They are described here:
MeteoSince2000.htm
and 1-hourly data are downloadable from here:
Mean01A-final.zip
(...) First however have a look at appended two ASCII files,
of which one contains the pointing measurements and the other a small
source catalogue with source names and J2000 equatorial coordinates
(RA and Dec in degrees) only.
The pointing data are those Roman Feiler has extracted for me from
a larger data set, except that at the outset I removed obviously
corrupt records and tagged some records with a # at the line beginning.
These come only from Autumn 2006. Many columns in it are not useful.
Here is a list of columns (an asterix marks data of interest)
1. source, *
2. fname,
3. az, *
4. el, *
5. elOff, *
6. elAmpl,
7. azOff, *
8. azAmpl,
9. azNoff,
10. azNAmpl,
11. src2cal,
12. cal,
13. data&time, *
14. naz,
15. nel.
The offsets from demanded positions (col. 5 and 7) were obtained
by a fit to cross-scans so that the coordinates are not reliable.
This worried me once, but soon I found that these coordinates are
really not so important for me, for (1) the offsets do not change
rapidly, (2) I know the demanded Az and El as determined by telescope
location and time. So in fact in my calculations I do not use them
at all and would like to warn you against possible troubles if you
would rely on them too heavily. The measured offsets are in degrees.
(...)
|
Subject: RE: 32m pointing
From: "Patrick Wallace"
Date: Th, February 11, 2010 23:38
To: "'Kazimierz Borkowski'"
(...)
> This dependence on weather might be significant at lower
> elevations, so if you think so, please do write back a few
> words about this.
The following table shows the amount of refraction at Torun for
three different sets of conditions:
Refraction at Torun, 30 GHz
Weather 1 = 1000.0 hPa -10.0 C 30.0 %
Weather 2 = 1000.0 hPa +30.0 C 90.0 %
Weather 3 = 950.0 hPa +30.0 C 90.0 %
el 1 2 3
90.00 0.00 0.00 0.00
85.00 1.50 2.07 2.00
80.00 3.02 4.16 4.03
75.00 4.59 6.33 6.13
70.00 6.23 8.59 8.33
65.00 7.98 11.01 10.67
60.00 9.88 13.63 13.21
55.00 11.98 16.53 16.01
50.00 14.36 19.80 19.19
45.00 17.10 23.59 22.86
40.00 20.37 28.09 27.23
35.00 24.39 33.64 32.60
30.00 29.54 40.75 39.49
25.00 36.50 50.36 48.81
20.00 46.59 64.29 62.31
15.00 62.77 86.69 84.02
10.00 93.32 129.11 125.18
9.00 102.98 142.59 138.27
8.00 114.69 158.97 154.17
7.00 129.14 179.26 173.88
6.00 147.34 204.95 198.85
5.00 170.81 238.35 231.35
4.00 201.94 283.18 275.02
3.00 244.48 345.61 335.96
2.00 304.56 436.42 424.85
1.00 392.12 574.96 561.04
deg mdeg mdeg mdeg
Subject: RE: 32m pointing
From: "Patrick Wallace"
Date: Su, February 14, 2010 10:44
Hi Kaz,
I have transformed your pointing file into a form that TPOINT
can understand, and have some reasonably convincing modelling
results to show you. The all-sky pointing I get is about
6 mdeg RMS. Of course, only part of this is the antenna
performance itself; the figure is a combination of three
things:
1) The known deficiencies of the 32m TCS (that it neglects
aberration, UT1-UTC and refraction) affect the logged
pointing data. On the basis of the one spot check we did,
my simulation of the 32m TCS errors is incomplete at about
the 2 mdeg level. There is in addition the fact that I
have used fixed values for pressure, temperature and
humidity.
2) The noise in the individual observations; i.e. what scatter
you would see if you repeated the same observation several
times.
3) The innate repeatability of the 32m antenna (i.e. the
ultimate performance limitation).
In the future, (1) can be eliminated by installing improved
algorithms in the TCS, and from the look of the residuals I'd
expect (2) to be at least as large as (3). With improved
absolute pointing, so that your cross scans start with the
source already centred to a fraction of a beamwidth, I would
expect (2) to decrease significantly.
Notes on the data conversion process:
* Although I have made provision for UT1-UTC when predicting
the true direction, the observations happen to be from a
period of a few weeks when UT1-UTC was small and changed
by less than 0.1s (from 0.15s on 2006/9/22 to 0.06s on
2006/12/12). I have therefore ignored it, for now.
* I had to interpret your "elevation offsets" as "zenith
distance offsets", in order to comply with your stated
encoder-minus-sky sign convention.
When I let TPOINT do a hands-off automatic fit, it produced
the following model (unit is arcsec):
coeff value sigma
1 IA -55.31 11.497
2 IE +96.58 3.233
3 & HASA -10.85 2.504
4 & HACA +14.64 1.381
5 & HASA2 +41.55 1.289
6 & HACA2 +31.39 1.177
7 & HASA3 +11.00 1.202
8 & HACA4 -6.15 1.273
9 & HASA5 -5.16 1.189
10 & HACA6 -5.34 1.187
11 & HACA8 +3.18 1.224
12 & HECA +9.09 2.424
13 & HESA2 +16.18 0.836
14 & HESE2 -40.98 3.376
15 & HECA2 +7.30 0.820
16 & HESA3 +1.90 0.804
17 & HECA3 -10.19 0.845
18 NPAE +129.11 11.193
19 CA -152.68 15.266
20 & HSCE8 +5.68 1.063
21 AN -5.42 2.286
22 AW +9.29 0.783
23 TF -101.37 2.800
Sky RMS = 22.67
Popn SD = 22.83
The usual geometrical model (zero points, nonperps, tilt) is the
set of terms IA IE NPAE CA AN AW, and TF is Hooke's-law flexure.
The rest are harmonic terms that TPOINT thought were
statistically significant and which presumably are trying to map
out various irregularities in bearings etc.
TPOINT decided that 73 of the 1672 stars were not consistent
with the model it found. They are marked in red on the first
plot, fit1.jpg (attached). This is TPOINT's "nine favourite
plots for an altaz" graph, and displays the pointing residuals
in various ways. For example, the top left plot is left-right
error versus azimuth, and the top middle is elevation error
against elevation.
With the outliers removed, the details become clearer - see
fit2.jpg. The jagged patterns are I think what you saw in your
own analyses. I got a slight improvement by manually adding a
17th-harmonic in azimuth affecting the az/el nonperp:
coeff value sigma
1 IA -53.36 11.275
2 IE +96.66 3.170
3 & HASA -11.11 2.458
4 & HACA +13.87 1.358
5 & HASA2 +41.54 1.266
6 & HACA2 +31.66 1.155
7 & HASA3 +10.92 1.182
8 & HACA4 -7.03 1.254
9 & HASA5 -4.24 1.173
10 & HACA6 -5.58 1.168
11 & HACA8 +3.10 1.200
12 & HECA +9.61 2.380
13 & HESA2 +16.19 0.820
14 & HESE2 -41.02 3.310
15 & HECA2 +7.29 0.804
16 & HESA3 +1.90 0.788
17 & HECA3 -10.17 0.828
18 NPAE +132.25 10.984
19 CA -155.65 14.972
20 & HSCE8 +5.88 1.042
21 AN -5.95 2.245
22 AW +9.43 0.768
23 TF -101.29 2.745
24 HVSA14 -5.91 1.130
25 HVCA14 -6.77 1.119
Sky RMS = 22.22
Popn SD = 22.40
The revised residuals are shown in fit3.jpg. I'm sure further
improvements are possible.
It's instructive to plot the total pointing correction,
unpolluted by observation noise. The final attachment, fit4.jpg,
shows this, after the zero points have been arbitrarily fitted in
order to improve the presentation.
Subject: RE: 32m pointing
From: "Patrick Wallace"
Date: Mo, February 15, 2010 15:20
Dear Kaz,
(...)
By trial and error, I have found that multiplying your azOff
figures by sin(El) seems to give a dAz value that is much more
plausible. If I empirically introduce this sin(El) scaling, and
if I ration myself to 13 coefficients, I get the following TPOINT
model, with only 16 observations rejected:
coeff value sigma
1 IA -141.40 8.729
2 IE +226.19 11.419
3 & HASA2 +24.58 1.190
4 & HACA2 +17.54 1.132
5 & HESE -122.07 8.415
6 & HECA +11.50 1.408
7 & HESA2 +16.30 0.820
8 & HECA3 -10.09 0.839
9 NPAE -134.80 8.496
10 CA +126.01 11.482
11 AN -7.63 1.157
12 AW +6.09 0.673
13 TF +18.42 8.740
Sky RMS = 23.00
Popn SD = 23.09
> (the covariance matrix tells me there are very, very strong
> correlations).
Here are the correlations using the above model. Most of the
trouble is always the set of terms affecting azimuth as a
function of elevation: the Az/El nonperp (NPAE) and boresight/El
nonperp (CA) are highly correlated with each other and with the
Az index error (IA).
IE -0.0052
HASA2 -0.0300 +0.0033
HACA2 +0.1614 -0.0003 -0.0220
HESE +0.0041 -0.9858 -0.0027 +0.0002
HECA +0.0887 +0.0051 -0.0642 +0.0083 -0.0172
HESA2 -0.0040 +0.0357 +0.0029 -0.0003 -0.0418 -0.0410
HECA3 -0.0037 +0.1696 +0.0023 -0.0003 -0.1568 -0.0571 +0.0336
NPAE +0.9496 -0.0043 -0.0316 +0.2079 +0.0035 +0.0535 -0.0029 -0.0028
CA -0.9863 +0.0049 +0.0273 -0.1905 -0.0039 -0.0723 +0.0034 +0.0033 -0.9859
AN -0.1049 +0.0053 +0.0763 -0.0099 -0.0043 -0.8243 +0.0038 +0.0034 -0.0629 +0.0855
AW -0.0616 +0.0854 +0.0385 -0.0051 -0.0688 -0.0887 +0.0664 +0.0589 -0.0444 +0.0528 +0.0583
TF -0.0073 +0.9864 +0.0040 -0.0006 -0.9495 -0.0116 +0.0382 +0.1814 -0.0061 +0.0068 +0.0064 +0.1020
IA IE HASA2 HACA2 HESE HECA HESA2 HECA3 NPAE CA AN AW
> If you did not correct the measurements for refraction, it got
> absorbed by your model.
My data file contains two sets of Az,El. The first is the
properly computed observed direction, which includes refraction
(albeit for average conditions). The second is my prediction of
your TCS's imperfect results, plus the offsets, i.e. the demanded
coordinates that would have centred on the source. The model
should therefore not contain a refraction component, and indeed
there is no TX term (ZD correction proportional to tan ZD).
Patrick Wallace
|
|
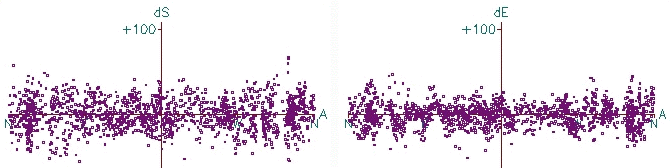
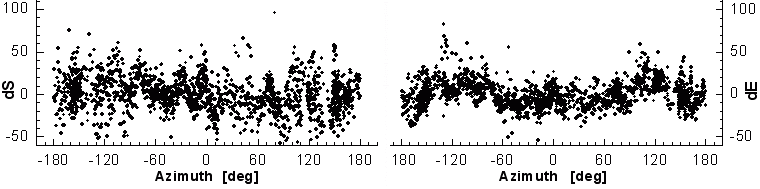
Comparison of residuals from 25-parameter fit by Patrick (top; taken from
fit3.jpg) and residuals from fitting of 13-parameter
Model4c (bottom). Left-right residuals (dS = -dAz)
are in left panels and elevation residuals are in right panels (dE = -dZD),
all scaled in arcseconds.
|
Subject: RE: 32m pointing
From: "Kazimierz Borkowski"
Date: Fr, February 19, 2010 15:05
Dear Patrick and Others,
(...)
Ever since you, Patrick, suggested that our AzOff measurements
might be somehow scaled (for they do not show significant
increase in the scatter towards the zenith), the idea kept
nagging me. After completing other urgent works I set
to reanalyse the data and my fitting of the pointing model.
First of all I must assure you that the azimuth offsets
are NOT scaled in any way - they represent offsets just
as they are measured by the azimuth counter. This assurance
comes from Roman who knows best.
Why then they do not exhibit this 1/cosEl pattern?
I am inclined to believe it may be due to combination of
1. large nonrandom errors that are independent of El, and
2. natural properties of such measurements.
In view of this, I would like to hear from you whether
in your practice you have whenever seen such pattern
in measurements or residuals in real data from other
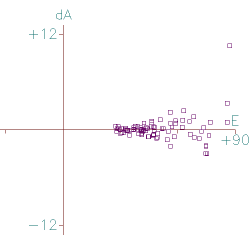
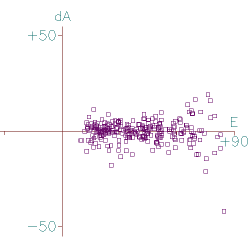
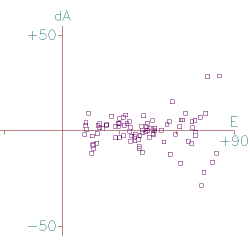
telescopes. I mean the pattern outlined by the red points
in the appended picture (which shows the azimuth residuals
from our most recent pointing Model4c).
I also tried to compare your fit with what I am obtaining
with my fitting program, especially with respect to
the final errors as represented by rms. There are number
of things that must be taken into account before any
reasonable comparison. I gave you the number 17.8" that
after scaling by sqrt(2) becomes 25", which is slightly
worse than your 23". This number (17.8) is however one
I obtained directly from the LSQ routine along with other
variances/sigmas. However, the data are supplied to
the routine along with individual weights for each
measurement (all equal to 1 for Elev and 1/cosEl for
Az offsets). Accordingly the sigma is a 'weighted sigma'.
Now I somewhat modified my programs so as to get also
the vector rms with and without scalings and weightings.
It was interesting to see how various approaches differ
in terms of final results. Here is a table summarizing what
I obtained. The first line is from old results (Model4c',
from larger set of data, not recalculated now) only for
reference.
(all numbers in arcseconds)
Sigma VRMSc RMSc(Az) RMS(El) RMS(Az) RMS VRMS
Model4c' 21.2 15.8
Model4c 17.82 27.10 15.54 11.21 24.17 26.64 37.67
Equal weights 24.24 27.66 22.95 12.12 22.95 25.95 36.70
Err*cos(El) 45.96 28.85 15.47 13.31 22.82 26.41 36.35
AzOff/cos(El) 25.52 38.49 24.80 11.22 55.16 56.29 79.60
Scaling AzOff*Sin(El) (Patrick's idea)
Az weighted 15.90 21.92 10.79 11.13 19.89 22.80 32.24
Az weights=1 20.91 22.70 10.72 11.95 18.11 21.70 30.69
Patrick's 23.00
The 'Sigma' column is what I obtain from the fitting
routine (weighted 3310 measurements), VRMSc and VRMS are
rms according to your 'vector' approach (as if there were
1655 'sky offsets', direction neglected), the first one is
with Az residuals scaled by cos(El) and the other (VRMS)
without scaling. Remaining colums contain rms calculated
the usual way (no vector treatment, no cosine scaling
except for RMSc(Az)). The rows correspond to:
Model4c - exact reproduction of the old analysis,
Equal weights - as above but all weights equal to 1,
Err*cos(El) - Az data weighted by cos(El),
AzOff/cos(El) - Az data scaled by 1/cos(El)
Az weighted - Az data scaled by sin(El) and weighted cos(El)
Az weights=1 - as above but weighted 1.
The last two cases could probably be compared to your fit
that had rms=23".
As is seen from the table, your and mine fits in this case
have almost the same 'vector' rms. And it is much smaller
than in Model4c or similar one without weighting (where it
is 27 to 28"), which evidently is the result of scaling
by sine(elevation). Of course, this scaling (by sinEl)
should not be used in practice but it is all right for
comparison purposes.
Considering the lack of apparent cosine pattern makes me
think that the weighting of Az offsets I have used in
producing the Model4c is unjustified. Without it we would
obtain somewhat different lookup table but in terms of
rms really not much different from the one we have presently
(as seen in the second and third line, the last two colums,
of the above table).
Therefore, I would be happy if you, Patrick or any one of you,
had a comment especially in this respect.
|
|

Expected error pattern (red dots) in postfit residuals
of azimuth offsets (black dots)
|
|
|
Subject: |
TPoint and RT32 |
|
From: |
"Kazimierz Borkowski" |
|
Date: |
Th, March 4, 2010 15:50 |
Dear Peter and All,
One of Action items of 9 Feb 2010 telecon was to give Patrick Wallace
some Torun pointing data for his inspection and analysis. On this we had
rather intense exchange of mails between February 10 and 22. Here is
written summary that I was trying to present during the last telecon a few
days ago. (...)
Patrick has analysed RT32 data of September - December 2006, exact
same as those used earlier to produce the lookup table
Model4c, the one
that is in use since 2007. The data consisted of some 1650 pairs of raw
dAz,dZD offsets, i.e. the differences between measured coordinates and
those demanded. The demanded coordinates used by the telescope control
system (TCS) were just precessed catalogue coordinates transformed to the
azimuth and zenith distance (without lookup table, without accounting for
effects of nutation, aberration, refraction, UT1-UTC difference or polar
motion).
Using his TPOINT application Patrick has performed fit of a 13-coefficient
pointing model to this data set. Prior to fitting, the data were modified
so as to account for the unaccounted effects and subjected to additional
scaling of azimuth offsets by sin(Elev). He arrived at RMS 'sky residuals'
of 23.0". By the sky residual is meant the vector sum of Az_res*cos(Elev)
and ZD_res.
For comparison, I have reconstructed my analysis, now 3 years old, to
obtain the Model4c using the original data. Then I scaled the azimuth offsets
by sin(Elev) to work on the same data as Patrick did, and fitted the same
model as used originally (to obtain the Model4c) to find RMS sky residuals
of 21.9 - 22.7" (the smaller number corresponds to the weighted fit I
normally do, the other is for unweighted azimuth data, that probably
correspond better to what Patrick did).
Conclusions:
1. TPoint model fits to RT32 data practically equally well as does Torun model
(both models being not very different in fact)
2. Patrick noted that our offset data do not show expected 1/cos(Elev) pattern
(see these examples)
3. Patrick demonstrated that in general a pointing can be improved (by a few arcseconds)
by introducing corrections for weather conditions to the mean refraction.
Recommendations for the new TCS regarding computation of demanded positions:
1. Include corrections for aberration
2. Include nutation
3. Include weather dependent refraction
4. Include UT1-UT predictions (and possibly polar motion)
Cheers,
Kaz |