|
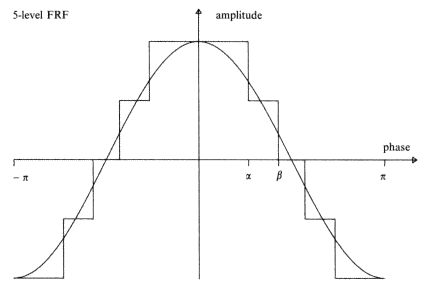
Fig. 1. One cycle of a 5-level fringe rotation function superimposed on the cosine function of the same period. |
(Letter to the Editor)
KAZIMIERZ M. BORKOWSKI
Toruń Radio Astronomy Observatory, Nicolaus Copernicus University,
Toruń, Poland
(Received 24 July, 1986)
| Abstract. This not new problem is analysed in view of discrepancies met in the literature. Practical formulae for calculation of the signal-to-noise ratio losses caused by quantization of the lobe rotation function in digital VLBI correlators are derived. Examples are given for a few simpler rotation schemes. |
Signals recorded using the very long baseline interferometry (VLBI) technique, after
correlation exhibit relatively high rates of interference fringes. This prevents direct
integration of normally very weak signals. Therefore, a fringe counter-rotation system
is built-in as an integral part of a VLBI correlator. It enables to almost entirely stop the
fringes by conversion of their frequency. The stopping is performed through a multiplication
of one of the two input signals to be correlated (or, equivalently, the correlation
product) by a fringe rotation function (hereafter referred to as FRF) which is a rough
approximation to a sinusoid whose frequency is set at a predicted rate of the fringes.
The approximation, itself justified by economy and efficiency of digital electronics
employed, leads necessarily to some loss of available signal. The loss is usually being
ascribed to dissipation of signal by the Fourier harmonics present in the imperfect FRF
used (e.g., Moran, 1976; Rogers et al., 1980; Kawaguchi, 1983). Although perhaps
intuitively appealing to many, such an assertion is undoubtedly not obvious.
The problem must have certainly been studied and solved by the pioneers of the VLBI technique but, to the best of my knowledge, have never reached the literature. This may explain at least a part of misleading enunciations published now and then in internal reports (e.g., Rayhrer et al., 1978; for criticism of this report see Borkowski, 1986) and in open literature (e.g., Fridman, 1983). In particular, the latter case is of real moment since the Fridman's paper in its essence was subsequently reprinted as a part of a book (Gubanov et al., 1983). This important publication received wide attention and good references (e.g., Fedorov, 1985), and as a bestseller is likely to be followed by new editions. It would not be difficult to demonstrate that the Fridman's formula for signal-to-noise ratio (SNR) loss due to quantization of the FRF is wrong and not just a misprint, but instead I shall derive a more general expression for the SNR loss, which validates (provided certain conditions are met) the earlier-mentioned relation to the harmonic content of the FRF.
To start with, assume a deterministic signal r is buried deeply in noise n and we wish to detect it by multiplying the data stream, xi = ri + ni, by a suitable function f (soon to be recognized as the FRF) and the averaging the product
| (1) |
| (2) |
If samples ni are statistically-independent, then the covariance E(ninj) vanishes always except for i = j, for which it becomes the variance of a single sample σi2, and the double sum in (2) reduces to Σ(fiσi)2.
In VLBI, the data samples are a product of two infinitely clipped signals, such that xi2 = 1 (hence, also E(xi2) = 1). Moreover, the correlation is usually so weak that all the σi can be safely set to 1 and the ri approximated by a rocos(φi – φo), where ro and φo are constant over integration interval of phase φ. As a matter of fact, the VLBI covariances do not wholly diminish to zero, nevertheless we shall keep the assumption of total independence of noise samples in order to arrive at the desired elegant solution. It should be born in mind, however, that the partial coherence of the band-limited noise, or its non-zero autocorrelation function, is the weakest point in the offered derivation. On the other hand it will be noted that though the coherence does significantly influence the SNR it affects in approximately the same degree the SNRs obtained with different FRFs, thus approximately cancels out in formulae for the relative loss factor.
Given the above assumptions conceming the VLBI practice, Equation (2) reduces to
| (3) |
The proportionality here is of course another approximation, but obviously very good one in view of a large number of fringe cycles normally averaged.
Dividing now Equation (3) by √(π)cosφo, which is the right-hand side of (3) evaluated for f(φ) = cosφ, we obtain the SNR loss factor of desired form
|
(4) |
Note that this expression is independent of φo, which can be interpreted to mean that the losses in both the orthogonal channels of the complex correlator, i.e., for f(φ) and f(π/2 – φ), are the same. Since the FRFs have always cosine or sine symmetries the formula (4) can be further simplified by limiting the integrations to the 0 – π/2 range of phase (instead of –π to π) and reducing the resulting coefficients. It was written intentionally in this form in order to clearly display its relation to the Fourier harmonics of the FRF. The numerator there is just the amplitude of the first harmonic, and the expression in the square brackets can easily be shown to be the sum of all the squared harmonic amplitudes. In spite of the fact that the discussed formula was derived under number of assumptions it proves very useful and convenient in analyses or practical VLBI correlator designs.
 | |
|
To have a few examples consider an FRF with 5 quantization levels as
illustrated in Figure 1. In the first quarter of its cycle the highest
level of our FRF extends up to α, from
α to β the
level weight is half that of the highest and for the remaining range
of phase, above β up to
π/2, the correlator is being effectively
inhibited. Substituting this FRF for f(φ)
in Equation (4) gives
| (5) |
which has a maximum of 0.9868 at α = 0.84074 rad and β = 1.34662 rad. This means that the optimal 5-level FRF introduces SNR losses of about 1.3% as compared to the sine wave FRF. An FRF most commonly used in practice is the 3-level approximation to a sinusoid, which is a square wave (the sign function of the sinusoid) zeroed over a π – 2α range of phase centered at each of its zero-crossings. It corresponds to setting β = α in the 5-level FRF, thus for its loss factor we have L(α,β) = 2 sinα/√(πα), which has a maximum of 0.9605 (hence, the 4% loss in SNR repeatedly, but not uniquely, cited in literature) at α = 1.16556 rad (in practice α is chosen to be the round 3π/8 = 1.178 rad with only negligibly small associated rise of the losses in SNR). The formula (5) may be used in a similar way for analyses of other simple FRFs by appropriate maneuvring the parameters α and β. Thus, in particular, L(0.8916,π/2) = 0.9737 for the optimal 4-level FRF and L(π/2,π/2) = 0.9003 for the 2-level FRF.
This work was partly financially supported through the Polish Government research problem RPB No. RR.I.11/2.
Borkowski, K. M.: 1986, Acta Astron., submitted [vol. 37, 89].
Fedorov, E. P.: 1985. Kinemat. fiz. nebesn. tel 1, 94.
Fridman, P. A.: 1983, Astrofiz. Issled. 17, 95.
Gubanov, V. S., Finkel'shtejn, A. M., and Fridman, P. A.: 1983, Vvedenie v radioastrometriyu, Nauka, Moscou, p. 220.
Kawaguchi, N.: 1983, J. Radio Res. Lab. 30, 59.
Moran, l. M.: 1976, Meth. Experim. Phys. 12C, 174.
Rayhrer, B., Reid, M. J., and Shaffer, D. B.: 1978, NRAO Report.
Rogers, A. E. E. et al.: 1980, in Radio Interferometry Techniques for Geodesy, NASA SP 2115, p. 275.